Difference between revisions of "Problems"
| Line 1: | Line 1: | ||
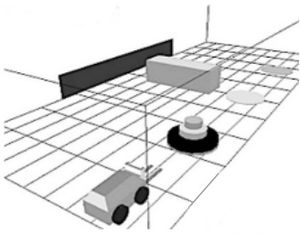
| − | + | == Problem 1 : Tower of Hanoi == | |
| + | [[File:bm1.jpg|problem 1|300px]] | ||
| − | + | ==== Description ==== | |
| + | Tower of Hanoi with mobile robots<ref>S. Cambon, R. Alami, and F. Gravot. A hybrid approach to intricate motion, manipulation and task planning. The Int. Journal of Robotics Research, 2009.</ref>. Work in progress: converting this problem to a humanoid robot setup. | ||
| − | == | + | ==== Challenge ==== |
| − | |||
| − | |||
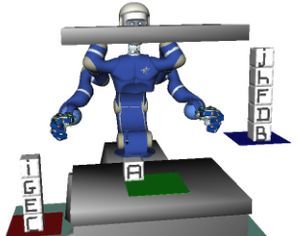
| − | == Blocks-World 3D == | + | == Problem 2 : Blocks-World 3D == |
[[File:bm2.jpg|problem 2|300px]] | [[File:bm2.jpg|problem 2|300px]] | ||
| + | |||
| + | ==== Description ==== | ||
| + | |||
| + | ==== Challenge ==== | ||
Blocks-World 3D with geometric constraint on the (mandatory) intermediate location. | Blocks-World 3D with geometric constraint on the (mandatory) intermediate location. | ||
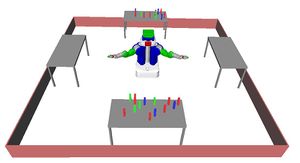
| − | == Rearrangement | + | == Problem 3 : Rearrangement Planning == |
[[File:bm3.jpg|problem 3|400px]] | [[File:bm3.jpg|problem 3|400px]] | ||
| + | |||
| + | ==== Description ==== | ||
| + | |||
| + | ==== Challenge ==== | ||
The rearrangement planning problem | The rearrangement planning problem | ||
| Line 20: | Line 28: | ||
<ref>F. Lagriffoul, B. Andres, ''Combining Task and Motion Planning: a culprit detection problem'', The International Journal of Robotics Research, 2016.</ref>. | <ref>F. Lagriffoul, B. Andres, ''Combining Task and Motion Planning: a culprit detection problem'', The International Journal of Robotics Research, 2016.</ref>. | ||
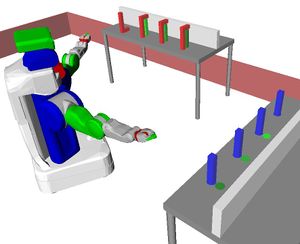
| + | == Problem 4 : Sorting Objects == | ||
| + | [[File:bm4.jpg|problem 4|300px]] | ||
| + | |||
| + | ==== Description ==== | ||
| + | The goal constraints are that all 7 blue blocks must be on the left table and all 7 green blocks must be on the right table. There are also 14 red blocks. | ||
| + | |||
| + | ==== Challenge ==== | ||
| + | The close proximity of the blocks forces the planner to carefully order its operations as well as to move red blocks out of the way. | ||
| + | |||
| + | == Problem 5 : Non-Monotonic == | ||
| + | [[File:bm5.jpg|problem 5|300px]] | ||
| + | |||
| + | ==== Description ==== | ||
| + | The robot must move the green blocks from the left table to a corresponding position on the right table. Both the initial and goal poses are blocked by four blue and cyan blocks respectively. | ||
| + | |||
| + | ==== Challenge ==== | ||
| + | Critically, the blue and cyan blocks have goal conditions to remain in their initial poses. This is the source of the nonmonotonicity as the robot must undo several goals by moving the blue and cyan blocks in order to solve the problem. | ||
== References == | == References == | ||
Revision as of 16:41, 13 January 2017
Contents
Problem 1 : Tower of Hanoi
Description
Tower of Hanoi with mobile robots[1]. Work in progress: converting this problem to a humanoid robot setup.
Challenge
Problem 2 : Blocks-World 3D
Description
Challenge
Blocks-World 3D with geometric constraint on the (mandatory) intermediate location.
Problem 3 : Rearrangement Planning
Description
Challenge
The rearrangement planning problem [2] [3].
Problem 4 : Sorting Objects
Description
The goal constraints are that all 7 blue blocks must be on the left table and all 7 green blocks must be on the right table. There are also 14 red blocks.
Challenge
The close proximity of the blocks forces the planner to carefully order its operations as well as to move red blocks out of the way.
Problem 5 : Non-Monotonic
Description
The robot must move the green blocks from the left table to a corresponding position on the right table. Both the initial and goal poses are blocked by four blue and cyan blocks respectively.
Challenge
Critically, the blue and cyan blocks have goal conditions to remain in their initial poses. This is the source of the nonmonotonicity as the robot must undo several goals by moving the blue and cyan blocks in order to solve the problem.
References
- ↑ S. Cambon, R. Alami, and F. Gravot. A hybrid approach to intricate motion, manipulation and task planning. The Int. Journal of Robotics Research, 2009.
- ↑ G. Havur et al., Geometric rearrangement of multiple movable objects on cluttered surfaces: A hybrid reasoning approach, Proceedings of ICRA 2014.
- ↑ F. Lagriffoul, B. Andres, Combining Task and Motion Planning: a culprit detection problem, The International Journal of Robotics Research, 2016.